Cosecant Calculator

The cosecant calculator helps you calculate the cosecant of an angle. Finding the value of csc() trigonometric function is now easy.

To use this csc calculator enter the angle value and choose from degrees or radians. Click the calculate cosecant button to see the result.

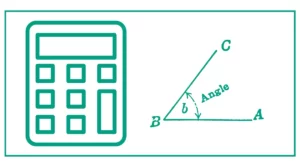
The cosecant of an angle in a right-angled triangle is defined as the length of the hypotenuse divided by the length of the side opposite the angle. It is also called the csc.

The formula of the cosecant is:

csc x = 1/sin x. csc x = Hypotenuse/Perpendicular

OR

csc x = 1/sin x. csc x = Hypotenuse/Opposite Side

You might want to calculate the foci or find the half angle.

Understanding the Cosecant Function

Before we dive into the specifics of the calculator, let’s review the cosecant function and its significance in trigonometry.

The cosecant, often abbreviated as csc, is one of the six fundamental trigonometric functions. It is defined as the reciprocal of the sine function. In mathematical terms:

csc(θ) = 1 / sin(θ)

where θ (theta) represents the angle in question.

In a right triangle, the cosecant of an angle is the ratio of the length of the hypotenuse to the length of the side opposite the angle. This relationship can be expressed as:

csc(θ) = hypotenuse / opposite side

Key Features of Our Cosecant Calculator

Our cosecant calculator is designed with user-friendliness and versatility in mind. Here are some of its key features:

1. Angle input in degrees or radians
2. Precise calculations with up to 8 decimal places
3. Instant results for quick reference
4. Simple and intuitive interface
5. Compatibility with various devices and browsers

How to Use the Cosecant Calculator

Using our cosecant calculator is straightforward. Follow these simple steps to find the cosecant of any angle:

1. Enter the angle value in the “Angle” input field.
2. Select the unit of measurement (degrees or radians) from the dropdown menu.
3. Click the “Calculate Cosecant” button.
4. The result will be displayed below the button, showing the cosecant value of the entered angle.

For example, if you want to find the cosecant of 30 degrees:

1. Enter “30” in the angle input field.
2. Select “Degrees” from the dropdown menu.
3. Click “Calculate Cosecant”.
4. The calculator will display: csc(30°) ≈ 2.00000000

This result means that the cosecant of 30 degrees is approximately 2.

Applications of the Cosecant Function

The cosecant function and our calculator have numerous practical applications in various fields:

1. Trigonometry: Cosecant is fundamental in solving trigonometric equations and identities.
2. Physics: It’s used in calculations involving waves, oscillations, and rotational motion.
3. Engineering: Cosecant is applied in structural analysis and electrical engineering.
4. Navigation: It plays a role in determining distances and angles in maritime and aerial navigation.
5. Computer Graphics: Cosecant is used in rendering and animation algorithms.

The Cosecant Graph and Its Properties

The graph of the cosecant function has several interesting properties that are worth noting:

1. Periodicity: The cosecant function repeats every 2π radians or 360 degrees.
2. Vertical asymptotes: The graph has vertical asymptotes at x = nπ, where n is any integer.
3. Range: The range of the cosecant function is (-∞, -1] ∪ [1, ∞).
4. Symmetry: The cosecant function is odd, meaning csc(-x) = -csc(x).

Inverse Cosecant: Arccosecant Function

While our calculator focuses on the cosecant function, it’s worth mentioning its inverse, the arccosecant or inverse cosecant function. This function, often written as arccsc or csc⁻¹, does the opposite of what our calculator does – it finds the angle when given a cosecant value.

The arccosecant is defined for all real numbers less than or equal to -1 and greater than or equal to 1. Its principal value range is [0, π/2) ∪ (π/2, π].

Cosecant Identities and Formulas

To fully leverage our cosecant calculator, it’s helpful to be familiar with some common cosecant identities and formulas:

1. Reciprocal Identity: csc(θ) = 1 / sin(θ)
2. Pythagorean Identity: csc²(θ) = 1 + cot²(θ)
3. Cofunction Identity: csc(θ) = sec(π/2 – θ)
4. Negative Angle Identity: csc(-θ) = -csc(θ)
5. Double Angle Formula: csc(2θ) = csc(θ)sec(θ) / 2

Practical Examples Using the Cosecant Calculator

Let’s explore two practical examples to demonstrate the use of our cosecant calculator in real-world scenarios.

Example 1: Engineering Application

An engineer is designing a support beam for a bridge. The beam forms a 60-degree angle with the horizontal, and the engineer needs to calculate the ratio of the beam’s length to its vertical rise. This ratio is the cosecant of the angle.

Using our calculator:
1. Enter “60” in the angle field.
2. Select “Degrees” as the unit.
3. Click “Calculate Cosecant”.

The result shows: csc(60°) ≈ 1.15470054

This means the beam’s length is approximately 1.15 times its vertical rise.

Example 2: Physics Problem

In a physics experiment, a pendulum’s motion is described by a sine function. The researcher needs to find the reciprocal of the sine at a specific point in the pendulum’s swing, which occurs at π/6 radians.

Using our calculator:
1. Enter “0.523598776” (π/6 in radians) in the angle field.
2. Select “Radians” as the unit.
3. Click “Calculate Cosecant”.

The result shows: csc(0.523598776 rad) ≈ 2.00000000

This value can be used in further calculations related to the pendulum’s motion and energy.

Tips for Using the Cosecant Calculator

To get the most out of our cosecant calculator, keep these tips in mind:

1. Always double-check your input, especially when switching between degrees and radians.
2. Remember that cosecant is undefined for angles where sine is zero (0°, 180°, 360°, etc.).
3. Use the calculator in conjunction with other trigonometric tools for comprehensive problem-solving.
4. Practice converting between degrees and radians to become more comfortable with both units.
5. Verify calculator results with approximate mental calculations when possible.

References

• 3 Cosecant, Secant, & Cotangent. (n.d.). 3 Cosecant, Secant, & Cotangent. webpages.uidaho.edu/learn/math/lessons/lesson03/3_03.html
• Cosecant Definition (Illustrated Mathematics Dictionary). (n.d.). Cosecant Definition (Illustrated Mathematics Dictionary). mathsisfun.com/definitions/cosecant.html